Foams Made out of Felt
A foam is a finite 2-dimensional CW-complex with extra properties. This one opening sentence is already more advanced than any of my usual blog posts. Let me define a finite 2-dimensional CW-complex in layman’s terms.
To construct such a CW-complex, we can start with a bunch of discrete points. This will be the 0-dimensional part of our future CW-complex. To continue, we glue-in segments between some pairs of points, making the whole thing into a 1-dimensional CW-complex. We can view such a complex as a graph. Now, what we have left to do is glue some disks in. There are two ways to do it. First, we can take the disk’s border and attach it to one of the points. Second, we can glue the border of the disc to a cycle in a graph.
The previous paragraph explained how to construct a finite 2-dimensional CW-complex. Foams have additional properties. Given a point in the CW-complex, its neighborhood needs to be homeomorphic to one of three objects:
- An open disc. Such points are called regular points. These are any points from inside of the discs.
- The product of a tripod and an open interval. Such points are called seam points. These are any points from inside of the segments. To meet the tripod condition, all the segments have to attach themselves to three discs.
- The cone over the 1-skeleton of a tetrahedron. Such points are called singular vertices. This means our starting vertices can’t remain unattached. The underlying 1-skeleton of our complex has to be a 4-regular graph (each vertex has to have degree 4). Moreover, any two edges coming from the same vertex must be on a disc’s border.
Some of the coolest foams are tricolorable. A foam is called tricolorable if it is possible to color its faces each in its own color by using three colors total so that at the seams, the faces of all three colors meet.
I first heard about foams during my brother Mikhail Khovanov’s lecture. He made a fascinating claim about tricolorable foams. If you remove regular points of a particular color from a foam, then the neighborhood of each point is an open disc. I was so curious, I decided to make a physical model. My readers know I hate crocheting, so I thought making a model out of felt would be easier. Thus, I made a tricolored neighborhood of a singular point. The two pictures show the same model from different angles.
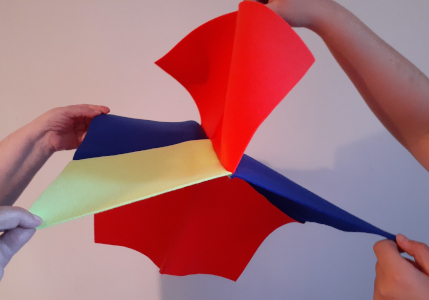
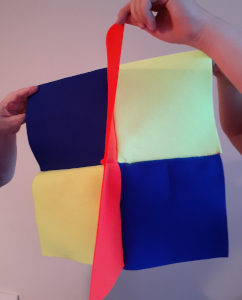
Then, I needed to check my brother’s statement and made the same model with one color attached to the foam by zippers. This way, I can actually unzip one color and see the result. This color in real life is neon-green but looks yellowish in the pictures.
Guess what? The result was a smooth neighborhood isomorphic to an open disc. My brother was right!
Share:
Double Maths First Thing: Issue A | The Aperiodical:
[…] third and final legend for this week: Tanya Khovanova is making foams out of felt. A foam is a mathematical object rather than something to make […]
13 November 2024, 4:39 am